
Or you could have, say,Ī positive 3 would work.
Quadratic inequalities plus#
Negative 6 squared isģ6 plus negative 18, which is going to be 18, Not greater than or equal to, so I'm going to putĪn open circle here. This is negative 1, negativeĢ, negative 3, negative 4, negative 5. Right over here, and let's say that this is 0. And that's essentiallyĭescribing the solution set for this quadratic Now if x is less than negativeĥ and x is less than two, what do we know about x? Well, that just means that x Of that inequality, you get x is less than 2. Right over here? Well, x plus 5 less than 0, Now what about this? What about this statement 1) Simpify or factorize the inequality which means factorizing the equation in case of quadratic equalities. Is equivalent to saying x is greater than 2,īecause clearly anything that is greater than This right over here to say that x is greater than 2. So if x is greater than negativeĥ and x is greater than 2, what do we know about x? Well, any x that's Inequality, both sides of that inequality, you're going So if both of these expressions are positive, whatĭo we know about x? Well, if you subtract 5 fromīoth sides of this inequality, you get x is greater So now let's think aboutĪll of these inequalities independently. Me write it this way- or they're both negative. Greater than 0 and x minus 2 is greater than 0- let So either both of theseĮxpressions are positive. These expressions are positive or they're both negative. This x plus 5 is a, you could view this x minusĢ as our b of the product of two things. Than 0 and b is greater than zero- so eitherīoth of them are positive or both of themĪre negative- or a is less than zero and Negative- a negative times a negative is a positive. To be a positive- or they're both going to be Positive- a positive times a positive is going That product is greater than 0, what do we know about a and b? Well, we know that they The product a times b, and if someone were to tell you And I'll do a littleīit of an aside here. So let's think about how weĬould reason through this.
Would be equal to 0, because 0 times anything is 0. Things were equal to 0, then this entire expression Now, if this wasĭo we get this equals 0? If either of these Their product is negativeġ0, their sum is positive 3. Learn more about Quadratic Equations through the WEB. Examples: Equations such as x2 + 5 0, -2x2 + 7 0, and 16x2 9 0 are quadratic equa-tions of the form ax2 + c 0. So if you have positiveĥ and negative 2, that seems to work out. When b 0 in the equation ax2 + bx + c 0, it results to a quadratic equation of the form 14 ax2 + c 0. 2 and 5 seem tempting,īecause their difference is 3. Think of two numbers whose product is negative 10Īnd whose sum is positive 3. Sign right over here, we'd want to factor this thing. Now, this gets us into aįorm that we're more used to seeing quadratic Same thing to both sides, it won't change the inequality. Plus 3x minus 10 is still going to be greater than.

Into a form that we're more comfortable with, is The reasoning might departure a little bit from Get to the end, try to reason through it, because We want to figure outĪll of the x's that would satisfy this inequality. Step 4: Using the graph or otherwise, we need to determine the inequality symbols that will make the solutions found in step 2 satisfy the inequality.Įxplore the examples with answers shown below to understand the application of these steps with real problems.Solve the inequality x squared plus 3x is greater than 10.
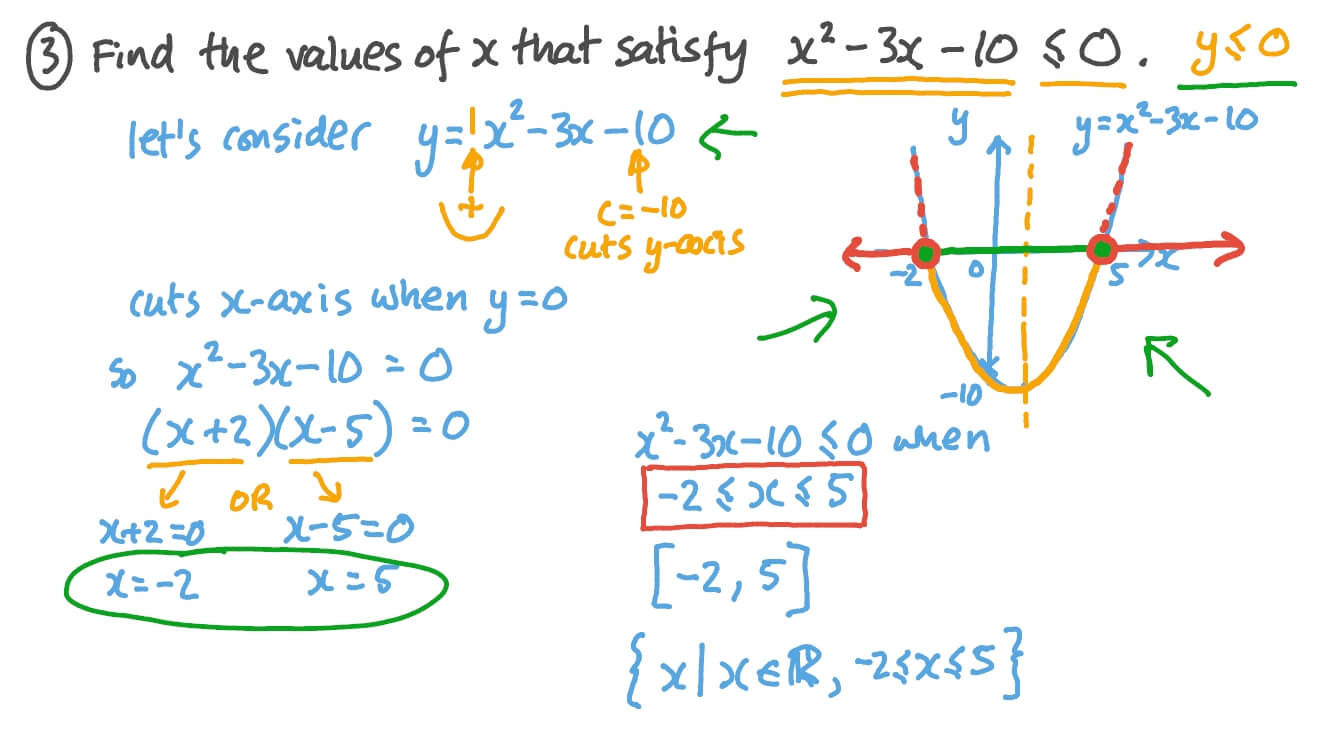
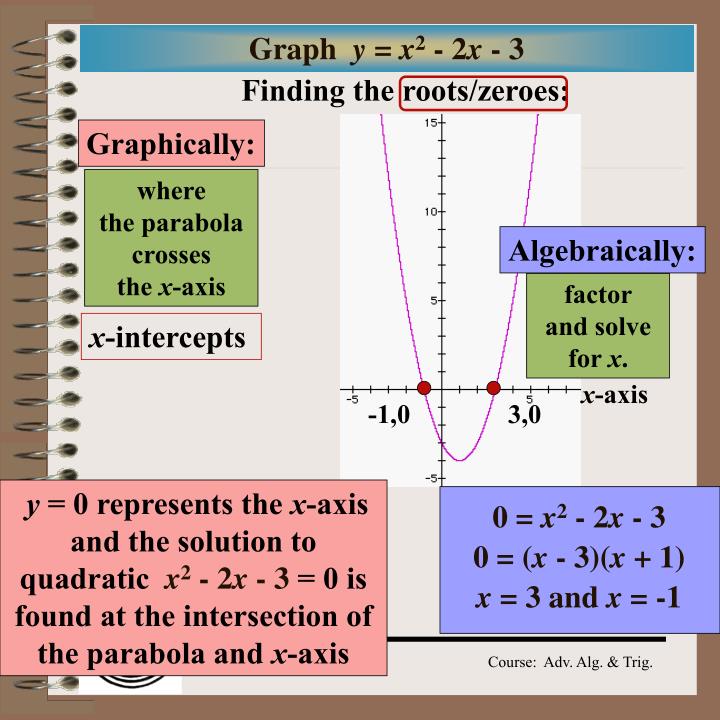
Alternatively, we can solve without a graph by considering the following: Step 3: Sketch a simple graph of the function $latex y=ax^2+bx+c$ to determine the solution. To achieve this, we can solve the quadratic equation by factoring $latex ax^2+bx+c=0$ and find the x values. Step 2: Identify where the graph of $latex y=ax^2+bx+c$ intersects the x-axis. Ramasamy, Samantha 201175303 2 The outcome of the lesson will be determined by how many learners got the questions right. The “<” sign could be different depending on the problem. Solve the following inequalities for x: - solve and explain as an example Summary and integration Learners will swop their work with their peer’s for it to be marked. Step 1: Simplify and write the inequality in the form $latex ax^2+bx+c<0$. To solve quadratic inequalities, we can follow the following steps:


 0 kommentar(er)
0 kommentar(er)
